Figure 3: A screen shot from an e-Proof for the product rule for continuous functions.
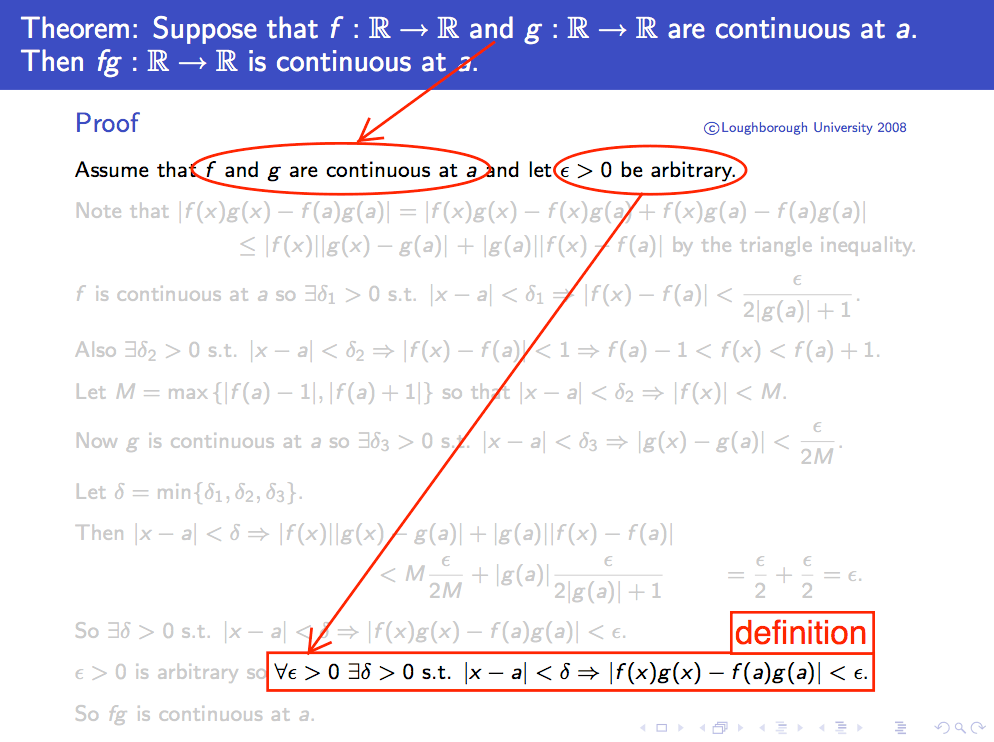
The accompanying audio for this screen says:
In the first line, we state our assumption that f and g are continuous at a, which corresponds to the premise of our theorem. We also let epsilon greater than zero be arbitrary, because we want to show that fg satisfies the definition of continuity at a, which we will achieve by the end of the proof. Doing so involves showing that something is true for all epsilon greater than zero, so choosing an arbitrary epsilon means that all our reasoning from now on will apply to any appropriate value.
© ISDDE 2011 - all rights reserved
Alcock, L., Wilkinson, N. (2011) e-Proofs: Design of a Resource to Support Proof Comprehension in Mathematics. Educational Designer, 1(4).
Retrieved from: http://www.educationaldesigner.org/ed/volume1/issue4/article14
Retrieved from: http://www.educationaldesigner.org/ed/volume1/issue4/article14